Disclaimer: Right so I have done this on this website already. Then something relatively minor changed and I started editing up the old version to fit the changes. Then something major changed and completely invalidated entire sections of the existing version. So rather than attempting to edit up the old version (which I will keep available here for full transparency sake) I decided to rewrite the thing. That said there is a lot of cross over in the early parts so it’s only really worth the read if you want to know my thoughts on how things should work. This particular text assumes no prior knowledge of RWBY and only contains Volume 2 spoilers. For a show with 6 seasons/volumes at time of wtiting and taking the overarching plot into consideration this isn’t too bad.

Table of contents

1. An Introduction to RWBY and Dust.

For the uninitiated RWBY is an online show made by the American company (Rooster teeth who I am not affiliated with) and takes place on a planet known as Remnant. Excluding a small number of cities most of Remnant is dominated by monsters referred to as Grimm. Due to the hostile nature of these Grimm technology on Remnant is different than it is on earth. Despite possessing enough technology to not only make guns but incorporated guns into almost any type of melee weapon, melee weapons are the primary effective tool against Grimm. Specifically, in the hands of trained fighters called Huntsmen (and huntresses). The four main settlements have an army and a police force but for the most part Huntsmen represent the main defensive force against the creatures of Grimm. In my personal opinion the main difference between Remnant and earth is not the ni on constant threat, but instead a resource called Dust.

Dust is important in RWBY. To the extent that we are given an info dump about dust before we even meet the main character. Dust is referred to as a “a naturally occurring energy propellent” and holds a very similar role to oil in our world. With some differences. The first is that Dust is crystalline in nature so where oil is pumped Dust is mined (making it closer to coal during the industrial revolution in this respect). On Earth 50% of the oil we use goes into fuels, but it also finds uses in plastics, medicine and clothing. On Remnant Dust comes in many different forms, each distinctly different.

The best way to describe the types of dust would be to think of an element system. Fire dust when used makes fire. Earth dust when used makes earth. Air dust when used makes wind. Water dust while confirmed to exist hasn’t actually been shown in the show but would assumedly make water. From here Dust can be combined “both artificially and naturally” into other types of dust. While some of these are expected, such as ice and steam, others are much more abstract such as gravity and hard light. On one hand I’m frustrated that there is no official list of all the dust types yet. On the other there is one thing I found particularly interesting about how Remnant treats dust.

“It was crazy. We have dust … but I mean there’s no such thing as magic.”

Remnant does not perceive the ability to manipulate gravity itself using the right coloured crystal to be magic. Maybe they did at one point in time but modern Remnant as we watch it in the show is technologically advanced enough that to them it isn’t magic. It’s a science.

Now there’s tonnes of interesting things when it comes to dust’s existence. From a sociological standpoint certainly but that isn’t my strong point. From a scientific standpoint Dust is ridiculous. For a quick reference gravity dust can break the speed of light if used correctly. That’s the level of reality breaking we are talking about here. The other thing is Remnant seems to be de-sensitised to this. They use the stuff everywhere. They have shops casually selling the stuff in bulk. But there is some reasoning. For starters it’s exceptionally convenient. No need to worry about large power plants to convert fuels into electricity, just use electricity dust. A part of me wonders if they discovered fire by rubbing together sticks or by using the red crystal first. No need to take drinking canteens on a trip, take water dust. The stuff you’ve packed for your trip still heavy? Gravity dust. So on and so forth. The second and main reason for this passage existing is that the sheer power held in Dust puts anything you’ll ever get your hands on to shame.

2. Getting started

Exactly how powerful is Dust. Well the short answer is, more powerful than anything you’ll likely get your hands on but ultimately it varies. While I could just say here’s, a number let’s compare it to real life examples you’d be taking my word on the number. So instead I am going to go through all the workings starting with the logic.

We are going to be calculating the energy density of ice dust? Why. Well the first reason is that it is the most commonly used dust in RWBY by far. The second is that it is possible to get a lot of the numbers from the same episode and the third is that this is not the first time I’ve calculated the energy density of ice dust. This will be the fourth time. With more information avaliable than the previous times but it also means that I can sanity check the numbers against the other times I have done this. With that said let us begin.

3. Ice dust

In season 2 episode 11  https://youtu.be/CUYhvPoxuas?t=478 (Episode link timestamped) we see Weiss (character in white) giving Blake (character in Black) a selection of dust Vials. This is arguably the best situation for determining the energy capacity of any dust type as from the previous link we can determine the dust’s volume and from later on in the same episode (Here: https://youtu.be/CUYhvPoxuas?t=611 ) we see the only fight where Blake uses any significant amount of Dust as of time of writing. It doesn’t matter which of the colour of dust ice dust actually is as all the vials are the same size. Which leads quite nicely into part a.

3a) Determining the volume of Ice dust used.

Luckily for the purposes of this particular part of the maths most of the hard work is already done. A 1:1 3D model of Gambol Shroud (Blake’s weapon) already exists https://www.myminifactory.com/object/3d-print-gambol-shroud-rwby-20990 so it is simply a measure of opening up the model in some 3D software and making an educated guess as to how far in the pistol mechanism an ammo clip goes.

Estimated Ammo clip dimensions: 122.97*22.842*13.063 (mm)

Aside: The aforementioned 3d model is made for the purposes of 3d printing and most 3d printers only have an accuracy of 0.2 when printed. 

Next task is to determine how many dust vials would fit in Blakes’s ammo clip. While @8:01 would imply that there are 6 dust vials in the clip a few instants earlier shows that each dust vial is 29.5 pixels wide and 85 pixels worth of un-used space. Meaning that Blake’s ammo clip could have held 8 dust vials. Some simple maths later we obtain

Dust Vial dimensions approximate: 15.37*22.842*13.063 (mm)

This however has two issues. The first is “what shape are the dust vials?” This is simple enough to answer. Cylinders. Bullet’s are cylindrical, the dust is going into a gun this simply makes sense. Which poses a problem as according to this same math the 15.37 and the 13.063 are both diameters. However as the measurement of 15.37 was simply an estimated depth of how far into a gun a magazine goes ammo clip rather than a direct measurement and a 2mm deviation between that and the actual measurement isn’t too big in the greater scheme of things. As for the second the second dimension in the above measurement needs to be lessened slightly as so to make space for the walls of the clip itself. Unfortunately there is no good angle to get this so any reductions are estimates at best. Even worse looking at the lengths of 13mm calibre bullets doesn’t help in this matter as the smallest length bullet of this size, the 56 Spencer has a case of 39.24. (This doesn’t invalidate the firearm’s of remnant entirely as it will be later proved that dust is far more powerful than modern propellants.) The best that we can estimate about this is to take the measurement’s from a real magazine https://www.bevfitchett.us/ar15-m16-2/images/3125_12_161-lower-receiver-dimensions.jpg and assume that this magazine has to have walls of similar thickness.

22.842-(0.1*2) = 22.642

Dust Vial Dimensions Final: 13*22.6*13 (mm)

From here it is only a question of simple math to obtain the total volume of the dust canister:

\pi \times (\frac{13}{2})^2 \times 22.6 = 2.99975 ml \approx 3ml

For reference a medical teaspoon is 5ml.

Aside: What about the dust container itself?

This is an interesting conundrum in and of itself. Dust normally coming in three forms, cut crystals, uncut crystals and powdered crystals. There are also ways of interpreting what the container itself is. Either the canister is glass in which case the dust must be powdered (as dust crystals are repeatedly shown to be hexagonal) or the canister follows a standardised colour coding, in which case it is impossible to tell how much dust is inside as the canister itself could be housing empty space inside it. Fortunately due to the amount of times we see these canisters throughout the show, of various sizes and how the colours match the cut/uncut crystals exactly in other scenes we could assume glass of negligible thickness or we could consider that powdered dust could be compressed into pellets before use and therefore would retain it’s shape without a canister to hold it.

3b) Determining the volume of ice generated

What happens to the dust is incredibly simple to explain with words. But rather difficult to describe with numbers. Blake, using dust, effectively creates an ice statue replica of herself. Here is where thing’s get a bit more complex. Fortunately, we have a value for Blake’s height, alongside all other characters found in this season of RWBY from a height chart. https://vignette.wikia.nocookie.net/rwby/images/f/f1/Sizechart.jpg/revision/latest?cb=20140913091405 A minor issue with this is that in this image Blake is wearing heels and a bow, something quite easily rectified. The real problem is that for the calculation we don’t need Blake’s height. We need Blake’s volume or more specifically we need the volume of the ice statue Blake makes of herself. This means Blake, her hair, her weapon and her clothes and so on.

i] The Volume of Gambol Shroud (Blake’s weapon).

This is thankfully a rather simple process of opening up the 1:1 model of Gambol Shroud, piece by piece and simply reading off the volume before adding them together being careful not to count duplicates (as so to only make one Gambol Shroud)

97174+27707.9+127695+38047.3+26415.2+207126+183167+346.834+43308.5+45319.1

796306.834mm^3

796.306834 ml

ii] Blake’s Volume

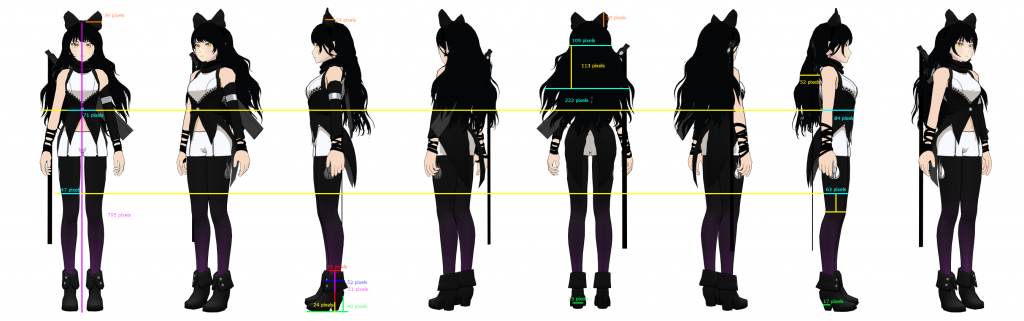
While not immediately apparent as to why we are now going to make various measurements of Blake’s body. Doing this all at once so that all the measurements are in the same place. Blake’s height from toe to head (in heels) is 1.68 meters. In a character reference this same length is also 795 pixels. After some working out where Blake’s leg enters the heels we find that they add an additional 24 pixels to her height which corresponds to:

Added height: \frac{168}{795} \times 24 = 5cm

Blake’s height: 163cm

Two more required measurements require simple single measurements that only require a little maths to determine and Blake’s abdominal circumference and Thigh circumference:

Taken at the measurement of the belly button (which is approximately the height of the elbow)

Minor axis (across front of chest): \frac{168}{795} \times 71 = 15cm

Assuming arms are relaxed and the back straight the backs of the arms align with the back of the back:

Major axis: (side view of stomach): \frac{168}{795} \times 84 = 17.75cm

Circumference of an ellipse: \pi \times \sqrt{2 \times (\frac{a}{2})^2 + (\frac{b}{2})^2}

Abdominal circumference: 51.62cm

Thigh measurements taken at 10cm above the knee

10cm = 47 pixels

Minor axis (front view): \frac{168}{795} \times 47 = 9.9 cm

Major axis: (side view): \frac{168}{795} \times 63 pixels = 13.3cm

Thigh circumference: 36.8cm

Which leads us to why have we taken these measurements at the beginning of this section? We need Blake’s Volume and while it would be possible to transform Blake into a number of Cylinders and spheres not only would this require a lot more measurements than those above but has a rather wide margin for error. Instead we run off the assumption that humanoids on Remnant have approximately the same proportionality as humanoids on Earth. Which then Leads us to how do we measure the Volume of a human without putting them under water and measuring the displaced liquid. From: http://www.dtic.mil/dtic/tr/fulltext/u2/628948.pdf

HumanVolume = SurfaceArea \times 62.9 \times (\frac{Weight}{Height})^{0.578}

Simplifies this dramatically. Now we only need the Surface Area, Weight and Height of Blake. We already have Blake’s Height so the next question easiest of the three to measure is Weight. Only we can’t put a fictional character on a set of scales. Instead we have to rely on more research. This time from: https://digitalcommons.unl.edu/cgi/viewcontent.cgi?article=1006&context=usnavyresearch

FemaleWeight = -40.2 + 0.42 \times (AbdominalCircumference) + 1.3 \times (ThighCircumference)

Blake’s Weight = -40.2 + 0.42 \times 51.62 + 1.3 \times 36.8 = 29.3kg

Aside: This is not the first attempt of measuring weight. The first method used was this one (http://www.eurecom.fr/en/publication/3189/download/mm-publi-3189.pdf) which while more accurate is not suitable for measurements under 35kg. This also reveals that Blake is severely underweight, practically anorexic. (BMI around 11) Moreover Blake is hardly the odd one out in this situation. There are of course two ways of looking at this. First is that the assumption that humanoids on Remnant and Earth are proportional is completely wrong. Most likely due to the animation style of RWBY and the fact that animation in general has a way of giving females unnatural proportions in the first place. The second way of looking at it is that this is in fact true. For starters Remnant isn’t earth. An example of this is that gravity on Remnant is roughly Two thirds of that on earth: https://www.reddit.com/r/RWBY/comments/65gkbt/how_much_gravity_does_remnant_have/

\frac{6.43}{9.81} = 0.655

Even then the characters in RWBY repeatedly propel themselves with gun recoil, jump incredible distances, perform impossible feats of climbing up falling debris. Blake repeatedly has the ability to pull herself around with a piece of ribbon. Sure pulling herself around at the speeds she does could be considered a feat of strength more but the fact the ribbon is more than capable of carrying her weight is more impressive than it should be. The ribbon being made of an interesting material in and of it’s own rite, channelling aura through weapons having an impressive effect on it’s durability or Blake (and for that matter everyone) is really light weight. Sure this is circumstantial at best but the alternative is to assume Blake has the same build as others her age and making the assumption that she is the same as the average weight for her age and gender here on earth (which as a trained fighter is unlikely).

The last requirement for volume is Body surface area which thankfully is simply substituting numbers into equations if height and weight are known. From https://www.calculator.net/body-surface-area-calculator.html?csex=f&bodyweight=29.3&bodyweightunit=kilogram&bodyheightfeet=&bodyheightinch=&bodyheight=163&x=123&y=15 and averaging.

Surface Area: 1.16m^2

This finally giving way to:

Volume = 1.16 \times 62.9 \times (\frac{29.3}{163})^{0.578}

Blake Volume = 27 L

iii] Volume of everything else

All we have left is to determine the volume of the rest of Blake’s constituent outfit and other yet unmeasured volume. All we can really do for this is take measurements, assume shapes for each part.

The main addition of volume from the shoes come from both the heels and the effective “collar” around the ankle. The heel is the easier of these two so starting with that one:

Assuming the heel is a cuboid: (It’s not. See the aside)

40*25*17 pixels

(\frac{168}{795} \times 40) \times ( \frac{168}{795} \times 25) \times ( \frac{168}{795} \times 17)

( \frac{168}{795}^3) \times 40 \times 25 \times 17

160.43 cm^3

As for the shoes collar assuming that it’s a tube (cylinder with a cylinder taken out of it):

Pixels: 52 diameter – 29 diameter and 51 height

Cm: 10.99 diameter – 6.13 diameter and 10.78 height

Cylinder:

(\pi \times (\frac{10.99}{2})^2 \times 10.78) - (\pi \times (\frac{6.13}{2})^2 \times 10.78)

2816.82 cm^3

So for a single shoe:

704.21 cm^3

For both shoes:

1408.41 cm^3

Next for the bow.

Assuming one side to be a triangle based pyramid.

39*24*38 pixels

\frac{1}{3} \times \frac{1}{2} \times (\frac{168}{795} \times 39) \times ( \frac{168}{795} \times 24) \times ( \frac{168}{795} \times 38)

\frac{1}{6} \times (\frac{168}{795})^3 \times 39 \times 24 \times 38

55.94cm^3

For entire bow:

111.88 cm^3

Finally for the hair:

Assuming a trapezium based prism

Pixels: (109 vs 222) * 113 * 52

(\frac{1}{2} (\frac{168}{795}) \times (109+222)) \times (\frac{168}{795} \times 113) \times ( \frac{168}{795} \times 52)

(\frac{168}{795})^3 \times \frac{109+222}{2} \times 113 \times 52

9177.12 cm^3

Aside: Why not clothes?

The first and main reason for not including clothes in this calculation is the fact that Blake’s clothes are for the most part rather form fitting and do little to add volume to Blake’s ice statue. The only exception to this would be the two pieces of fabric that look like they come from a penguin tail tuxedo. As to why these aren’t included the answer is that from the above calculations above are almost certainly an overestimation of volume and the heels of the shoes due to treating the typically semi-elliptical prism as a cuboid leads to an overestimation of volume. Similar arguments can be made by each part calculated through assumed shapes above. By neglecting the volume added to the statue from this fabric it is hoped that the overestimation of these can be offset somewhat.

iv] Total volume generated

Now it’s just a matter of adding everything up to get the final total volume of the ice statue:

796.31+27000+160.43+ 1408.41 +111.88+9177.12 = 38696.72 ml

Quick note: 1ml = 1cm^3

After this point we have a small assumption to make in that the ice statue is solid. This assumption is justified for two reasons. For starters Roman (the person Blake fights in this scene) struggles to retrieve his weapon from the statue so any thickness the statue does have, is sufficiently thick to not break under a little force. The second reason we can make this assumption is because we actually see the statue being broken a few moments later and as far as I can tell from going through screenshots of the debris the only hollow pieces are the frozen pieces of clothing which would have had frozen Blake’s body pieces in them. This does add an interesting fact in that we would have of overestimated the volume of ice given the statue was accurate enough to leave air gaps between clothes and the main body had we calculated the volume of the clothes as an additional layer over Blakes’s body (which was the initial plan).

3c) How to create ice?

Now we do not have any official in show explaination as to how ice duct creates ice. The company that makes RWBY however has another series where they go into the science of fictional characters fighting one another. As a part of this they have fought their own companies characters against those from other universes. Now here’s a quote from one such episode:

“Obviously that much ice doesn’t fit into two vials of Dust. She’s likely using… Fusion energy and Vaporization energy on the Nitrogen in the air.”

Now two thing’s. First this means that ice Dust doesn’t make ice but rather solid nitrogen. Second this is genuinly insane. There is a whole host of reasons I don’t like this. But it’s made by the same company so this is basically as close as we are getting to an official mechnisim for making “ice” from ice dust so we can run the numbers from this.

We need to have 38696.72ml of solid nitrogen. Solid nitrogen (in bulk) has a density of 1026.5 kg/m^3 so through simple math:

1026.5 kg/m^3 \times 38696.72ml = 39.7221831kg

I am also going to assume that the nitrogen in the train car is at room temprature and pressure to begin with. Which seems reasonable enough. Nitrogen has a specific gas constant of 296.80 J/kgK so to cool from room temperature to the required temperature for liquid nitrogen (From 300K to 77.36K would require:

296.80 J/kgK \times 39.7221831kg \times (77.36 K- 300K)

-2,624,824.06 J

Now there is a quite obvious problem with this. That is that negative energy isn’t a thing. You can’t just subtract energy from an object, physics doesn’t work like that. Thankfully heat pumps, and by extention refrigerators, exist. Assuming that Dust is the best heat pump possible (for simplicity sake) the cooling efficiency of a heat pump is given by:

\frac{T_C}{T_H - T_C}

Where T_C is the temprature of the cold object and T_H is the temprature of the hot object. Now I am going to assume T_H remains at room temprature throughout the entire process. Mainly because if I didn’t the entire process of freezing the Blake sized statue would also increase the temprature of the air inside the train car by 16,191 Kelvin (15,917 celcius, 28,684 farenheit, over three times hotter than the surface of the sun) (see appendix). Now technically speaking I would have to find the value for all the values of cooling efficiency for T_C between 300K and 77.36K. I don’t feel like doing the working for intergals so thankfully computers are a thing that can do this step for me.

Average cooling efficiency: 200.545 (Yes heat pumps are one of the few things that can be over 100% efficient)

So with some more math:

\frac{2,624,824.06}{200.545} = 13088.45 J

But we have (exceptionally cold) gaseous nitrogen. We still need to make it a Solid. But first we need to make it a liquid. Using the latent heat of Vaporization:

199 kJ/Kg \times 36.14kg = -7,191,860 J

And the efficiency:

\frac{77.36}{300-77.36} = 0.34746

So:

\frac{7,191,860}{0.34746} = 20,697,979.7J

Now we need to cool our liquid nitrogen even further to solid nitrogen temperatures (from 77.36K to 63K). The specific heat of liquid nitrogen: 57107.95 J/(kg*mol*K) where Where mol = 28.01 so:

57107.95 \times 28.01 \times 39.7221831 \times (63-77.36) = -912425109.406J

Average cooling efficiency: 0.305794

So:

\frac{912425109.406}{0.305794} = 2,983,790,098.58 J

Now we need to turn our liquid nitrogen into solid nitrogen. The latent energy of fusion of nitrogen is 25.7 KJ/kg. So:

25.7kj/kg \times 39.7221831kg = -1,020,860.11 J

And an efficiency of:

\frac{63}{300-63} = 0.26582

Leading too:

\frac{1,020,860.11}{0.26582} = 3,840,378.5 J

And adding everything together:

13,088.45 J + 20,697,979.7 J + 2,983,790,098.58 J + 3,840,378.5 J = 3,008,341,545 J

3d) The Properties of Solid Nitrogen

Now there is one more thing to talk about here. Under normal circumstances, having turned nitrogen into a solid you would conclude that this is the energy required for ice Dust to make “ice”. There is however a little more to talk about here. At 63 Kelvin Solid Nitrogen does not act like ice. This intuitively makes sense in the fact the two are not the same material but there is a little more to it than that. At 63 Kelvin Solid nitrogen doesn’t shatter like ice does and like it is shown to do so in the show but rather bends and deform’s more like metal. Solid Nitrogen is also transparent at 63 Kelvin. Now while we do see transparent “ice” formed in the show from time to time for the most part the “ice” formed is white. Now in reality ice is white due to air trapped in the solid itself. Seeming as we are freezing the air itself however (and seeming as the other constituent parts of air will have long frozen before the nitrogen) we can’t really rely on the same mechanics happening here. There is however a reason I’m being very particular about mentioning the properties of Solid Nitrogen at 63 Kelvin. That is because as the temprature of solid nitrogen changes so does it’s physical properties. It starts shattering (like ice) at 30 Kelvin and becomes white at 20 Kelvin (which coicedently give it about the same structural resilience as ice at around this temprature). This means to make solid nitrogen analogous to ice it needs to be cooled even further to around 20 Kelvin. With the Specific heat capacity of solid nitrogen being 1.6 kJ/kgK:

1.6 kJ/kg K \times 39.7221831kg \times (20K-63K) = -2730000J

Average cooling efficiency: 0.16323

\frac{2730000}{0.16323} = 16,743,000 J

And so finally (with some rounding) the actual energy required is:

3,008,341,545 J+ 16,743,000 J = 3,025,000,000 J

4) Translation into real world comparasons.

In words over 3 Billion Joules of energy came out of the ammount of dust you could fit on a teaspoon with room to spare. To actually draw a comparason to other fuels it is important to get the energy density of Dust which is quite simply:

3,025,000,000 J / 2.99975 ml = 1,008,400 MJ/L

But what does this number actually mean. Assuming the other forms of dust have approximately the same energy density if you replaced a AA battery with the same sized piece of lightning dust it would last 233,426 times longer. Assuming you could fill a petrol car on dust instead the same sized tank would go 29,485 times further. If you could digest dust a single teaspoon of dust that would cover the average man’s daily recomended intake of calories, for 482 days. In the scene that inspired this analysis Weiss gave Blake the approximate equlivalent of 16,397 grenades. Indeed the only fuels we have on earth with a higher energy density are nuclear in nature and dust has a wide variety of advantages over nuclear fuels not only in it’s versatility but also in it’s safety. Where the safety standards around nuclear fuels highlight limiting exposure in Remnant they store dust in display cases, weave it into clothing and directly inject it into their own bodies. While on one hand it’s impressive that people on Remnant can handle having multiple years worth of food injected into their bodies all at once it also speaks volumes as to how safe it is handled properly.

Appendix) Warming up the train.

To make the statue of ice, assuming the temprature of the surrounding air doesn’t change requires 3,025,000,000 joules of energy. Now assuming the train car dimentions on Remnant are similar to those on earth we have a volume of at most:

18.52m \times 2.9m \times 3.96m = 212.683m^3

Now the heat capacity of air held at a constant volume is:

0.7171 \frac{kJ}{kg /times K}

And the density of air:

1.225 \frac{kg}{m^3}

Some simple maths later:

\frac{3,025,000,000J}{ 212.683m^3 \times 1.225 \frac{kg}{m^3} \times 0.7171 \frac{kJ}{kg /times K}} = 16,191K

Now in reality this wouldn’t be the case. It would be smaller. Not only does the heat capacity of air held at constant volume increase (although not significantly) with increasing temprature with the scale of temprature we are talking about here over the timescale we are talking about here the heat wouldn’t have time to disperse over the entirity of the cabin, especially with nitrogen from the air being used to create the “ice” statue. But still the creation of the ice statue would warm the rest of the train car to far beyond deadly levels.